What is the shortest distance from a point to a line segment? On determining this distance, two scenarios should be distinguished, as given in the picture below.

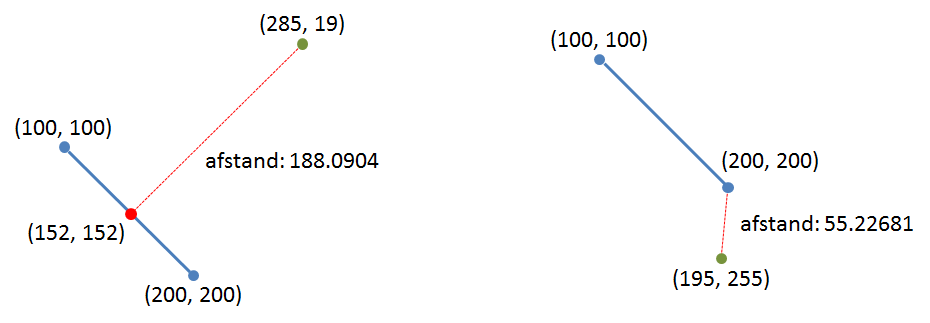
Assume that the line segment joins the points $$(x_1, y_1)$$ and $$(x_2, y_2)$$, and that we are searching for the shortest distance between this line segment and a point $$(x_3, y_3)$$. In the first scenario (left picture), the perpendicular line from the point $$(x_3, y_3)$$ intersects the straight line on which the line segment is situated in point $$(x_v, y_v)$$ on the line segment. This point is called the nadir. The shortest distance then equals the distance between the points $$(x_3, y_3)$$ and $$(x_v, y_v)$$. In the other scenario (right picture), the nadir is located outside the line segment, and the shortest distance is formed by the shortest distance from point $$(x_3, y_3)$$ to each end of the line segment $$(x_1, y_1)$$ and $$(x_2, y_2)$$.

Assignment

• Write a function distance to which four integers should be given. These numbers respectively represent the co-ordinates of two points $$(x_1, y_1)$$ and $$(x_2, y_2)$$. The function should return the Euclidean distance $$d$$ between those points, that is given by the formula \[ d = \sqrt{(x_1 - x_2)^2 + (y_1 - y_2)^2} \]

• Write a function nadir to which six integers should be given. These numbers respectively represent the coordinates of three points $$(x_1, y_1)$$, $$(x_2, y_2)$$ and $$(x_3, y_3)$$. The function should return two (real) co-ordinates $$(x_v, y_v)$$ of the nadir. The nadir is the intersection of the perpendicular from the point $$(x_3, y_3)$$ with the straight line through the points $$(x_1, y_1)$$ and $$(x_2, y_2)$$, and the line segment that joins the points $$(x_1, y_1)$$ and $$(x_2, y_2)$$. In order to do so, we first calculate the value \[ u = \frac{(x_3 - x1)(x_2 - x_1) + (y_3 - y_1)(y_2 - y_1)}{(x_2 - x_1)^2 + (y_2 - y_1)^2} \] The perpendicular line will only intersect the line segment if $$0 \leq u \leq 1$$. If that isn't the case, the function should return the value None twice for the coordinates of the nadir. If both ends of the line segment coincide, the denominator in the calculation of $$u$$, equals zero, but the nadir also coincides with the ends of the line segment. In other cases, the co-ordinates of the nadir are given by \[ \begin{eqnarray*} x_v &=& x_1 + u(x_2 - x_1) \\ y_v &=& y_1 + u(y_2 - y_1) \end{eqnarray*}\]

• Use the functions distance and nadir to write a function shortest_distance to which six integers should be given. These numbers respectively represent the co-ordinates of three points $$(x_1, y_1)$$, $$(x_2, y_2)$$ en $$(x_3, y_3)$$. The function should return the shortest distance between point $$(x_3, y_3)$$ and the line segment that joins the points $$(x_1, y_1)$$ and $$(x_2, y_2)$$.

Example

>>> distance(152, 152, 285, 19)
188.09040379562165
>>> distance(100, 100, 195, 255)
181.79658962697843
>>> distance(200, 200, 195, 255)
55.226805085936306

>>> nadir(100, 100, 200, 200, 285, 19)
(152.0, 152.0)
>>> nadir(100, 100, 200, 200, 195, 255)
(None, None)

>>> shortest_distance(100, 100, 200, 200, 285, 19)
188.09040379562165
>>> shortest_distance(100, 100, 200, 200, 195, 255)
55.226805085936306