Klaus Kemp¹ is the sole modern practitioner of a lost Victorian art form — arranging diatoms into tiny, dazzling patterns, like microscopic stained-glass windows.

Diatoms are single-celled algae that live in shells of glass-like silica. There are hundreds of thousands of varieties, ranging in size from 5 to 50 thousandths of a millimeter. In the latter part of the 19th century, professional microscopists arranged them into patterns for wealthy clients, but how they did this is unknown — they took their secrets with them. Kemp spent eight years perfecting his own technique, which involves arranging the shapes meticulously in a film of glue over a period of several days. In an article in Wired² he explained it as follows:

"As a youngster of 16 I had a great passion for natural history and came across a collection of sample tubes of diatoms from the Victorian era. I was immediately struck by the beauty and symmetry of diatoms. The symmetry and sculpturing on an organism that one cannot see with the naked eye astonished me, and after 60 years of following this passion I can still get excited from the next sample I receive or collect."

Assignment

In this assignment we ask ourselves the question how many smaller circles having radius $$r \in \mathbb{R}^+$$ fit inside a larger circle having radius $$R \in \mathbb{R}^+$$, without the smaller circles overlapping each other. For example, we can fit at most 12 smaller circles in the larger circle shown in the following figure.

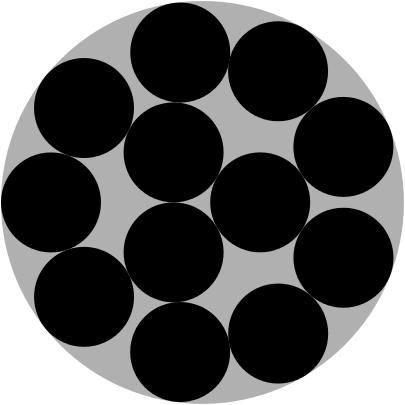
So far, nobody has been able to find the generic answer to this question, but the maximal number of smaller circles $$n$$ can be approximated using the following formula: \[n = \left\lfloor 0.83\frac{R^2}{r^2} - 1.9 \right\rfloor\] where $$\lfloor x\rfloor$$ is the largest integer that does not exceed $$x \in \mathbb{R}$$.

Input

Two numbers $$r$$ and $$R \in \mathbb{R}^+$$ — each on a separate line — that respectively indicate the radius of the smaller and larger circles. As such, it must hold that $$r \leq R$$ (you don't have check this explicitly in your code).

Output

A single line that indicates the maximum number of smaller circles that fit inside the larger circle, and the percentage of the larger circle that is covered by these smaller circles. Use the above formula to estimate the maximum number of smaller circles that fit inside the larger circle. The percentage of the larger circle covered must be expressed as a floating point number with two decimal digits. Rounding must be used to determine the digits of the floating point number.

Example

Input:

2.38
10.14

Output:

13 smaller circles cover 71.62% of the larger circle