The Collatz conjecture is a conjecture from number theory. It is based on a sequence of numbers — referred to as the hailstone sequence — that is constructed in the following way: take any integer $$n$$. If $$n$$ is even, divide it by 2 to get $$n / 2$$. If $$n$$ is odd, multiply it by 3 and add 1 to obtain $$3n + 1$$. The Collatz conjecture states that no matter what integer $$n$$ ($$n \geq 1$$) you start with, you will eventually always reach 1. This conjecture has been first proposed by Lothar Collatz in 1937. Up until now this conjecture has neither been proven nor rejected.

As an example, let's start from the number $$n = 12$$. The hailstone sequence that results from this number reads as follows:

12, 6, 3, 10, 5, 16, 8, 4, 2, 1

Based on this sequence it is said that the cycle length for $$n = 12$$ is equal to 10, because the length of the corresponding hailstone sequence (including the last value 1) is equal to 10. If we start from the number $$n = 15$$, we have a much longer hailstone sequence with cycle length 18:

15, 46, 23, 70, 35, 106, 53, 160, 80, 40, 20, 10, 5, 16, 8, 4, 2, 1

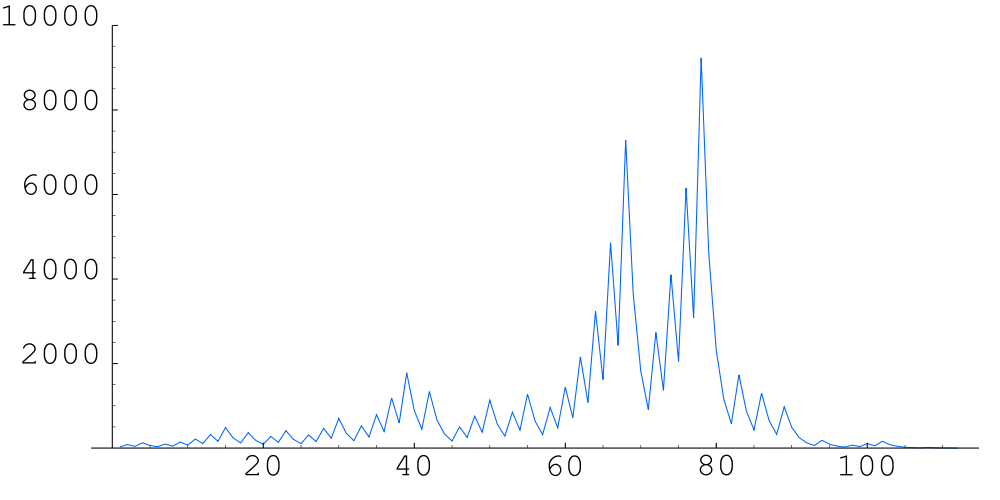
For $$n = 27$$ the cycle length is as high as 112, climbing up to a maximal value above 9000 before descending to 1:

27, 82, 41, 124, 62, 31, 94, 47, 142, 71, 214, 107, 322, 161, 484, 242, 121, 364, 182, 91, 274, 137, 412, 206, 103, 310, 155, 466, 233, 700, 350, 175, 526, 263, 790, 395, 1186, 593, 1780, 890, 445, 1336, 668, 334, 167, 502, 251, 754, 377, 1132, 566, 283, 850, 425, 1276, 638, 319, 958, 479, 1438, 719, 2158, 1079, 3238, 1619, 4858, 2429, 7288, 3644, 1822, 911, 2734, 1367, 4102, 2051, 6154, 3077, 9232, 4616, 2308, 1154, 577, 1732, 866, 433, 1300, 650, 325, 976, 488, 244, 122, 61, 184, 92, 46, 23, 70, 35, 106, 53, 160, 80, 40, 20, 10, 5, 16, 8, 4, 2, 1

The figure below illustrates the evolution of the hailstone sequence for $$n = 27$$.

Input

The input contains $$t$$ test cases ($$t \leq 100$$). The first line of the input contains the integer $$t$$. It is followed by $$t$$ lines that each contain an integer $$n$$ ($$n \geq 1$$).

Output

For each test case, print the cycle length for $$n$$ on a separate line.

Example

Input:

5
1
2
321
1111111111111
111111111111111111111111111111111111111111111111111111111111

Output:

1
2
25
261
1296